Series LRH/2 Code No. 30/2/1
MATHEMATICS
Time allowed : 3 hours Maximum Marks :80
sections - A, B, C and D. Section A comprises of ten questions of 1 mark each, Section B comprises of five questions of 2 marks each, Section C comprises of ten questions of 3 marks each and Section D comprises of five questions of 6 marks each.
SECTION A
1. The HCF of 45 and 105 is 15. Write their LCM.
2. If one zero of the polynomial 
, write the other zero.
3. A tangent PQ at a point P of a circle of radius 5 meets a line through the centre 0 at a point Q so that OQ = 13 cm. Find length PQ.
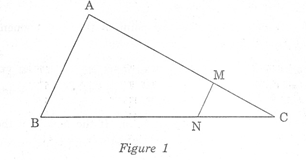
4. In Figure 1, MN II AB, BC = 7.5 cm, AM = 4 cm and MC = 2 cm. Find the length BN.
5. If 6x = sec Q and 
, find the value of 
.
6. Find the distance between the points, A(2a, 6a) and B(2a + 
, 5a).
7. If the sum of first m terms of an A.P. is 
+ 3m, then what is its second term ?
8. Find the value of k if P(4, -2) is the mid point of the line Segment joining the points A(5k, 3) and B(-k, -7).
9. The slant height of a frustum of a cone is 10 cm. If the height of the frustum is 8 cm then find the difference of the radii of its two circular ends
10. A die is thrown twice. What is the probability that the same number will come up either time ?
SECTION B
11. If -1 and 2 are two zeroes of the polynomial 
, find its third zero.
12. For what value of k will the following pair of linear equations have no solution ?
2x + 3y = 9; 6x + (k - 2)y = (3k - 2).
13. In an A.P., the first term is - 4, the last term is 29 and the sum of all its terms is 150.
Find its common difference.
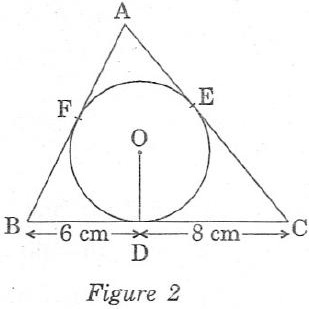
14. In Figure 2, a triangle ABC is drawn to circumscribe a circle of radius 3 cm, such that the segments BD and DC into which BC is divided by the point of contact D are of lengths 6 cm and 8 cm respectively. Find the side AB if the area of 
15. Without using trigonometric tables, find the value of the following :
cot Q . tan (90° - Q) sec (90° - Q) cosec Q + . tan 12° . tan 6Q° . tan 78°.
OR
Find the value of sec 45° geometrically.
SECTION C
16. Prove that 
is an irrational number.
17. Solve the following pair of linear equations for x and :
2 (ax - by) + (a + 4b) = 0; 2 (bx + ay) + (b - 4a) = 0
OR
A number consists of two digits. When the number is divided by the sum of its digits, the quotient is 7. If 27 is subtracted from the number, the digits interchange their places. Find the number.
18. The sum of first sixteen terms of an A.P. is 112 and the sum of its next fourteen terms is 518. Find the A.P.
19. In 
ABC, right-angled at A, BL and CM are the two medians. Prove that 
20. If tan Q + sin Q = in and tan Q - sin Q = n, show that 
OR
Show that : 
21. Draw a circle of radius 3 cm. From a point P, 7 cm away from the centre of the circle, draw two tangents to the circle. Also, measure the lengths of the tangents.
22. If point 
lies on the line segment joining the points A(3, -5) and B(-7, 9), then find the ratio in which P divides AB. Also find the value of y.
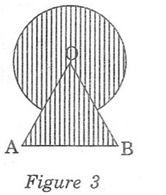
23. Find the area of the shaded region in Figure 3, where a circular arc of radius 7 cm has been drawn with vertex 0 of an equilateral triangle OAB, of side 12 cm, as centre.
OR
The rain-water collected on the roof of a building, of dimensions 22 m x 20 m, is drained into a cylindrical vessel having base diameter 2 m and height 3.5 m. If the vessel is full up to the brim, find the height of rain-water on the roof. 
24. Find the value of k for which the points A(9, k), B(4, -2) and C(3, -3) are collinear.
25. From a well-shuffled pack of playing cards, black jacks, black kings and black aces are removed. A card is then drawn at random from the pack. Find the probability of getting
(i) a red card,
(ii) not a diamond card.
SECTION D
26. Some students planned a picnic. The total budget for food was Rs. 2,000. But 5 students failed to attend the picnic and thus the cost of food for each member increased by Rs. 20. How many students attended the picnic and how much did each student pay for the food ?
OR
Solve the following equation for x: 
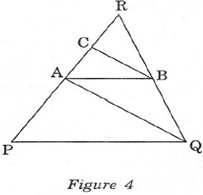
27. If a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, prove that the other two sides are divided in the same ratio. Using the above, do the following In Figure 4, PQ 11 AB and AQ II CB. Prove that 
28. From a window (9 m above the ground) of a house in a street, the angles of elevation and depression of the top and foot of another house on the opposite side of the street are 30° and 60° respectively. Find the height of the opposite house and the width of the street. [Use 
= 1.732]
OR
A vertical pedestal stands on the ground and is surmounted by a vertical flag staff of height 5 m. At a point on the ground the angles of elevation of the bottom and the top of the flag staff are 30° and
60° respectively. Find the height of the pedestal.
29. A container, open at the top, and made of a metal sheet, is in the form
of a frustum of a cone of height 24 cm with radii of its lower and upper ends as 7 cm and 14 cm respectively. Find the cost of milk which can completely fill the container at the rate of Rs. 25 per litre. Also find the area of the metal sheet used to make the container. 
30. If the mean of the following frequency distribution is 65.6, find the missing frequencies 
:
| Class | Frequency |
| 10-30 | 5 |
| 30-50 | 8 |
| 50-70 |  |
| 70-90 | 20 |
| 90-110 |  |
| 110-130 | 2 |
| Total | 50 |